In this article we will continue to focus on two branches of the Quadrivium, that of Number and Geometry.
We will begin with zero then we will move through the numbers one to ten exploring all related geometry along the way. Later articles go into great depth on number symbolism, the Platonic solids, Archimedean solids, Golden Ratio and Square roots. A series of construction lessons will be offered so those inclined can learn to create their own geometry and geometric patterns that can be used in a wide-range of activities.
We will begin this article with the ‘number’ zero, its history and symbolism.

Number – Zero
The information presented in this article is sourced from Robert Lawlor’s Sacred Geometry Philosophy & Practice unless otherwise noted.
Mathematical Concepts as a Basis of Thought
Mathematical concepts can be a prototype for:
- the dynamics of thought
- structuring and action
One & Zero: Unity & Nothingness
Robert Lawlor writes, “The starting point of ancient geometric thought is not a network of intellectual definitions or abstractions, but instead a meditation upon a metaphysical Unity, followed by an attempt to symbolize visually and to contemplate the pure, formal order which springs forth from this incomprehensible Oneness.”

“Ancient geometry begins with One, while modern mathematics and geometry begins with Zero.”
History of Zero
The concept of zero is a relatively new idea in history.
Its origins date back to 8th century AD in India.

During the century just before zero was introduced, a certain line of thought had been developing that mixed Hinduism (through Shankhara):

Adi Shankara with Disciples, by Raja Ravi Varma (1904)
and Buddhism (through Narayana):

A depiction of Lord Narayana at Badami cave temples
This line of thought emphasized the goal of obtaining personal transcendence and escape from karma through renunciation of the natural world.

It promoted mortification of the physical body. It was highly ascetic.

Its goal was the attainment of impersonal, blank void, a total cessation of movement within consciousness.

Interestingly, this time period is considered by many as a dark period in a long, rich spiritual heritage in India.
Lawlor states this time period was “a decline from the previous tradition which upheld a spiritual significance in both the manifested and the unmanifested expressions of God, and whose tantric and yogic practices worked towards an intensification of the relationship and harmonization between matter and spirit.”
This new philosophical perspective caused the concept of zero to take hold.
The concept was called ‘sunya‘ in Sanskrit, meaning empty.

Sunya became chiffra in Latin – meaning ‘null’ or ‘nothing’.
Simultaneously, ‘maya‘ took on new meaning.
Maya originally meant ‘the power to divide’ or ‘the dividing mind’. This original concept related to the cosmic creating process of how the Many came from the One, yet still existed in Oneness.
This is the concept of the individuation of Cosmic Consciousness, or the reflection of the Infinite Mirrors that create infinite individualized consciousnesses that are actually One consciousness.

During the time we are discussing, maya came to mean ‘illusion’ or the material aspect of the universe as illusion.
Within this change of definition physical reality came to be regarded as illusion, not-real, unimportant, or even worse: “bad”, “degenerate” or “evil”.

Culturally speaking, the reverse of this ‘spiritual nihilism’ of the East is ‘materialism’ of the West.

Western materialism began to emerge very strongly after the Industrial Revolution.
In the case of materialism – the polar opposite of ‘spiritual nihilism’ – the spiritual aspect of reality came to be seen as illusory, unimportant, not-real, or even worse “ridiculous”, “false”, “stupid”, “naïve” or “hindering”.

These two ideas are the extreme ends of the spectrum and neither are balanced ways of looking at reality.
Zero as a Notational Procedure vs. Zero as a Tangible Number
Babylonian, Ancient Greek and Mayan cultures used a symbol to denote an empty column when calculating quantities.
Aristotle and others ancient Greek teachers talked about the concept of zero philosophically but did not incorporate zero into their mathematics because their foundation was strongly built upon Pythagorean teaching in which the Monad was the ‘One’, meaning Unity. Unity was the source and foundation of reality, not zero, or nothingness.

Marking an empty column is only a notational procedure.
In Indian mathematics, zero is treated as a tangible entity, as a number.
For example, Indian mathematicians wrote (a x 0)/0 = a.
However zero was placed after 9, not before it, due to theological impulses. 1 was still seen spiritually at this point as the Infinite Unified Source – the ‘All’ or Foundation of Reality.
The Proliferation of Zero
Many Arabs in the 9th -14th centuries transmitted knowledge from the declining cultures of the east and Egypt to the rising cultures of Western Europe. This time period, which lasted from about the mid-eighth to mid-thirteenth centuries, was known as the ‘Islamic Golden Age’.
Zero was seen as a practical device for facilitating calculation and recording large numbers, particularly large numbers with an empty column.
Muhammad Ibn Musa Al-Khwarizmi (780-850) was a great 8th century mathematician often described as the father or founder of algebra.

He carried numerals with zero from India to the Islamic world.
Our word ‘algorithm‘ comes from his name.
“A half century after al-Khwarizmi, Abu Kamil Shuja ibn Aslam (850-930), an Islamic mathematician from Egypt, applied complex algebra to geometric problems, solving three non-linear equations fro three different variables…Abu Kamil was the first mathematician to employ irrational numbers as solution to quadratic equations, and his Kitab fi al-jabr wa al-muqabala (Book of Algebra), expanded on the work of Al-Khwarizmi.”1
In the 12th century Gerard of Cremona and Roberts of Chester translated Abu Kamil’s work into Latin. From there the use of zero then gradually moved into medieval Europe through Arabic settlements in Spain, the Indian numerals and zero coming along with it.

Though the use of zero seems inconsequential and innocuous to our modern minds, this fairly simple concept supported radical change in Western science and thought.
In the 16th century – the dawn of the Age of Reason – 0 was placed before 1, for the first time in history.
Placing zero before One suddenly allowed for the concept of negative numbers. Negative numbers are common to modern humanity, but they are illogical.
Interestingly, some monastic orders resisted, including the Cistercian Order (formed in 1098).
The Cistercians followed a mystical, Gnostic philosophy.

Saint Benedict and Saint Bernard (1542), by Diogo de Contreiras. Saint Bernard is depicted in the white habit of the Cistercians.
Their philosophy was the inspiration and foundation for the Gothic cathedral constructions in the Piscean Age. “Early Cistercian architecture shows a transition between Romanesque and Gothic architecture.”2

They claimed zero was a device of the devil.
This may seem silly to our modern minds, but they realized the profound implications zero would have on human consciousness that would open the door for humanity to separate itself from its spirituality and believe that the material reality was the only true reality.
Merchants adopted the zero, nonetheless, for ease of calculation.
Suddenly zero permitted numbers to represent ideas which have no form. This was an entirely new way of looking at numbers.
In antiquity, ‘idea‘ meant ‘form‘ and implied geometry and Idealism represented consciousness giving form to reality.
‘Idea‘, therefore, was redefined. Ideas became less real than physical ‘things’. Humanity began to become separated from the knowledge that consciousness (ideas/thoughts/emotions) formed all physical reality.

The ancients knew consciousness was the source of the physical. This began to slip away during the ‘Age of Reason’.
We could look back and mourn the loss of this knowledge, yet it was essential for the evolution of human consciousness to forget its source so that it could rediscover the knowledge in order to use it for higher purposes.
As humans, we are rediscovering that knowledge now.

Mathematical Consequences of Adopting the Zero
When zero was introduced, the additive basis of calculation had to be cast aside.
This created operations such as:
3 + 0 = 3
3 – 0 = 3
03 = 3
30 = 3 x 10
but 3 x 0 = 0
and 3 \ 0 = 0 (???)
Logic breaks down with multiplication and division of zero.
Illogical mathematics were nevertheless accepted due to convenience of quantitative operations.
Lawlor explains, “This breakdown of the simple, natural logic of the arithmetic structure allowed a complicated mental logic to take its place and invited into mathematics a whole range of numerical and symbolic entities, some of which have no verifiable concept or geometric form behind them.”

These included:
- relative numbers – negative quantities such as -3
- infinite decimal numbers
- algebraic irrational numbers – such as the cube root of 10
- transcendental irrational numbers – such as e, the basis of logarithms, which satisfy no rational algebraic equation
- imaginary numbers – such as square root of -1
- complex numbers – the sum of a real number and an imaginary number
- literal numbers – letters representing mathematical formulae
Unity was rejected. This led to a system requiring:
- complex interconnected hypotheses
- imaginary entities
- unknown x quantities which must be manipulated, quantified or equalized, as in the algebraic form of thought
Scientific Consequences of Adopting the Zero
Physicist Conrad Ranzan writes, “The rejection of aether and the consequential incomplete theory of gravity has led theorists to propose highly speculative universes of mathematical genre – abstractions devoid of reality.”
Dr. Harold Aspden concurs, “Modern physical theory has become abstract. The starting points of the original papers on the subject are mathematical, the treatment is mathematical and the conclusions are mathematical. In many instances there seems to be no relation whatsoever to the phenomena which make up the world of experimental physics.”
When studying science, particularly physics and cosmology, it is clear that we live in a mathematical universe – a mathematical abstraction.
Mathematical universes can be worked out on paper with equations, but they do not necessarily represent the real Universe.
Theoretical models can not necessarily be equated with physical reality.
As Nikola Tesla stated, “Today’s scientists have substituted mathematics for experiments, and they wander off through equation after equation, and eventually build a structure which has no relation to reality.”
Ranzan writes, “It all started with a geometric interpretation of gravity via a four-dimensional space-time. This so-called curved space interpretation became the foundation of the abstract mathematical universes. But since no one could say what was actually “curving” (what was behind the curvature relationship of space coordinates) the resulting cosmology was merely an abstraction.”
Aspden adds, “I wish to give my view that the mathematical theories of our universe, highlighted by Einstein’s Relativity, have given too much rein to the mathematician. His skills in providing one of the tools needed by the physicist have been set aside and he has tried to become a philosopher in his own right…superimposing a man-made vision of Nature and confusing us rather than recounting nature’s ordered structure with clear language.”
Dewey B Larson agrees. He tells us, “Closely connected with the use of obscure and incomprehensible concepts is the utilization of unusual and complicated methods of mathematical treatment. This device itself is perfectly legitimate. There are a multitude of applications in science and technology where the use of complex mathematics is essential to solution of the existing problems. But this is another tool which lends itself very readily to misuse, and there is a definite tendency in present-day practice to call upon complicated mathematical procedures as a means of forcing the observed facts into conformity with preconceived basic concepts, rather than adopting the logical but distasteful alternative of giving up these basic ideas that are erroneous or inadequate.”
Larson then adds, “The misuse of mathematics has not gone unrecognized. Lande tells us, for example, ‘The mathematical sign language with its complex symbols and non-commutative matrix algebra has become a veil shielding the simple meaning of the quantum laws from the scrutiny of common sense,’ and Bridgman calls our attention to the fact that the statistical methods may be used to ‘conceal a vast amount of actual ignorance.’
But the general tendency has been to glorify the complex and the abstruse. A liberal use of non-commutative mathematics, non-Euclidean geometry, and complicated statistical procedures has come to be regarded as the hallmark of erudition, and any publication, in the field of physics at least, which does not bristle with integral signs and complex equations is looked upon as lamentably deficient in scholarly quality, irrespective of the actual need for anything more than simple arithmetic.”
We will discuss cosmology and all of these points in an extensive series of articles which will begin after the Number/Geometry series.
Philosophical Consequences of Adopting the Zero
The idea of zero changed our ideas of philosophy & theology.
It changed our way of viewing nature.
It also changed our attitudes toward our own natures and the environment as a whole.
‘Western’ rationalistic mentality negated the ancient, revered concept of Unity.
Unity lost its position and became just another mere quantity.
Zero provided a framework for the development of atheism or negation of the spiritual.
It encouraged a mechanistic line of thinking in physics in the 19th century – an atomic theory where matter was composed of tiny building blocks, little spheres floating in a zero-empty void.

It encouraged the idea of separation between the quantitative and the non-quantitative.
The extreme of this idea suggests that everything which is non-quantitative is non-existent or zero.
In other words, everything that cannot be seen or measured (spiritual reality and consciousness) does not exist.
Psychological Consequences of Adopting the Zero
The concept of zero encouraged ideas of:
- the finality of death
- fear of death
- apathy; feelings of powerlessness, hopelessness, meaninglessness and worthlessness
- separation of heaven and earth
- separation of humanity from Nature
-
a range of existential philosophies based on the despair and absurdity of a world followed by non-being

The Concept of Zero & Nature
The concept of zero, or nothingness, creates a separation between our system of numerical symbols and the structure of the natural world.
Regardless of the complex theories humans can hold in their minds, from Nature’s view, zero does not exist.
Lawlor writes, “Everything remains here with us; the cycles of growth, utilization and decay are unbroken. There is no throw-away bottle.”


The Concept of Zero & The Belief in Separation
The introduction and utilization of zero allowed the concept of unity to become unthinkable.
Therefore, the idea of opposites strengthened.
“…Simply because in order for anything to be, to exist, it must, in the very positive affirmation of itself, negate that which it is not. Cold is only cold because it is the negation of heat. For a thing to be, its opposite must also be. There is then at the beginning of the created world a contingency of division of Unity into two.”

This belief in opposites – that is, opposing forces rather than merging tendencies – allowed the destructive ideas of elitism, racism, genocide, and political and religious viciousness to take root in the world and nearly destroy it.

Negation vs. Fractioning
Negation is the concept of zero discussed above – the concept of separation and nothingness.
After the adoption of Indian numerals and zero, the next step was the invention of negative numbers.
This created a number line that vanished in both directions.
Our current model:
…- 5, – 4, – 3, – 2, – 1, 0, 1, 2, 3, 4, 5…
gives a series of non-existent abstractions (negative quantities) which demand an absurd logic.
It requires complex algebra and trigonometry.
Negation leads to Imaginary Numbers
Negative numbers created a conundrum: square a negative number and it becomes positive.
There became an entirely new number line: the square roots of negative numbers: imaginary numbers (i)
i = √-1
Miranda Lundy comments, “Interestingly, it is the play between imaginary and real numbers that effortlessly produces the beautiful complexity of fractals and chaos theory, models of recursive shapes and processes we find all around us in nature.”
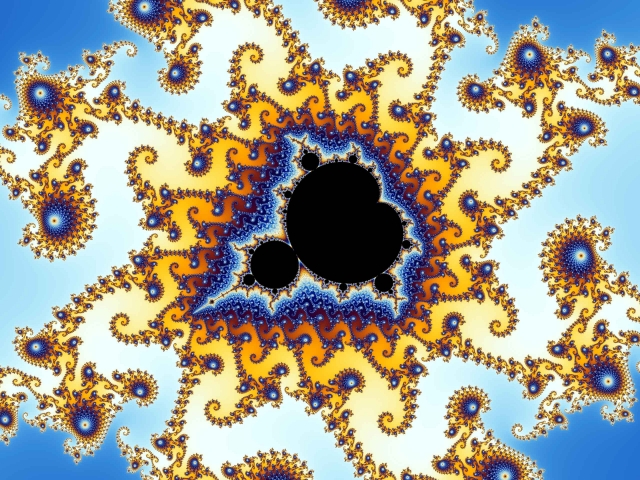
Fractioning
Everything that exists is a fraction of the unknown (Cosmic Consciousness).
The parts can be related to one another – therefore they are knowable.
The ancient Egyptian model:
…1/5, ¼, 1/3, ½, 1, 2, 3, 4, 5…
All the elements are natural and real.
They flow out from a central unity in accordance with the law of inversion or reciprocity.
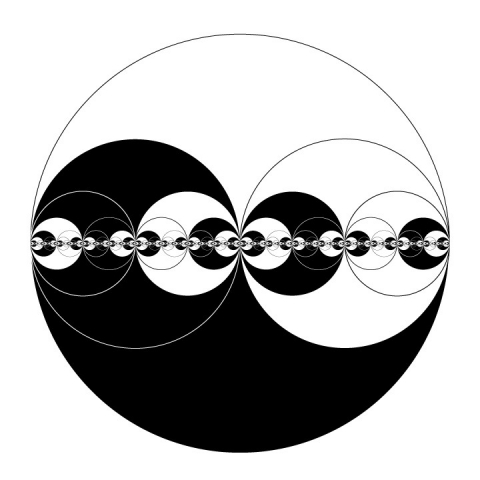
The essential law of Harmony is expressed by this series (covered in music section).
R.A. Schwaller de Lubicz writes, “The Number One is only definable through the number two: it is multiplicity which reveals unity…The intelligence of things exists only through what we may call an original fractioning and the comparison of these fractions to one another, which is then only an enumeration of the aspects of Unity.”
Inversion & Fractioning
The concepts of inversion and fractioning play a major role in physics:
- the Uncertainty Principle
- quantum entanglement
- concepts of the Black Hole
- the idea of continual interchange between matter and energy
- continual oscillation from the physical to metaphysical
These ideas encourage spiritual concepts such as:
- immortality of the soul
- rebirth and reincarnation
Robert Lawlor writes, “The progression of inverse (reciprocal) elements supplies a mental basis for the notion of perpetual interchange through reversal.”
Mathematical Axioms
Wikipedia tells us that “a statement or proposition that is regarded as being established, accepted, or self-evidently true” is an axiom.
That is, they are true because we say they are true.
Often with axioms we are not fitting our theories to any physical universe, but creating this universe ourselves.
Oftentimes, axioms declared to be true create paradoxes.
If so, the axiom can be changed to avoid the paradox, or the paradox can be ignored.
The following example is used to illustrate the state of modern mathematics and its inherent structure of separation.
Example: Axiom of Infinity
This axiom guarantees the existence of at least one infinite set.
On the Youtube channel Vsauce, there is an episode entitled, How to Count Past Infinity.
It illustrates an illogical way of reasoning that is fully accepted in mathematics today.
First we must define some terms:
Cardinal numbers – refers to an amount, any amount
Natural numbers – positive integers (whole numbers) and sometimes zero
Aleph-Null – the first ‘smallest infinity’ – refers to how many natural numbers there are, even numbers, odd numbers, rational numbers – bigger than any finite amount.
Here we run into the first paradox – there is only one ‘infinity’ – there is no smallest or largest infinity.
The mind games continue:
Ordinal Numbers – tells the position of something in a list, such as 1st, 2nd, etc.
Omega – comes after the infinite series of aleph null. It defines an order type.
Omega + 1 isn’t bigger than omega, it just comes after omega.
Power Set – the amount of subsets you can make from the original set (S) or the set of all subsets of S.
Power Set is 2 to the power of how many members the original set had (2S).
A Power set of natural numbers always comes up with ‘bigger infinities’.
The axiom of infinity says one set of infinite numbers exists.
The axiom schema of replacement says if you take a set, for example the set of all natural numbers, and replace each element with something else like tulips, what you are left with is also a set.
Take every ordinal up to omega and put omega + in front of each, you get omega + omega or omega x 2.
This allows us to construct new ordinals without end – Epsilon-naught.
The continuum hypothesis is biggest unanswered problem of this topic.
Replacement and repeated power sets keeps it going.
An inaccessible cardinal is one you can’t reach from below, it is so big.
Aleph-0 is inaccessible. Its existence is declared axiomatically.
The conceptual jump from nothing to the first infinity is like the conceptual jump from the first infinity to an inaccessible.
If this doesn’t make sense, there is a reason for that. It is illogical! It doesn’t make sense!
This complex and illogical way of thinking is accepted in mathematics – but only serves to create divisions in the minds of those who study these axioms.
There cannot be separate sets of infinites.
There can only be one infinity, and within this infinity lies all other infinite things.
Robert Lawlor tells us, “In terms of the orthodoxy of ancient mathematics, the symbols of mathematics should reflect the realities they describe.”

Creating these labels of aleph-null, omega, epsilon-naught…etc only muddies the waters and contributes to schisms of the mind.
Sri Aurobindo tells us in The Life Divine, “At the origin of things we are faced with an infinite containing a mass of unexplained finities; an indivisible full of endless divisions, an immutable teeming with mutations and differentiations, a cosmic paradox is at the beginning of all things. This paradox can only be explained as One, but this is an infinite Oneness which can contain the hundred and the thousand and the million and the billion and the trillion…This does not mean that the One is plural, or can be limited or described as the sum of the many. On the contrary, it can contain the infinite many because it exceeds all limitation or description by multiplicity, and exceeds at the same time all limitation by finite, conceptual oneness.”

- Meisner, Gary B., The Golden Ratio: The Divine Beauty of Mathematics, Race Point Publishing, 2018
- https://en.wikipedia.org/wiki/Cistercian_architecture
Recent Comments